5.2. Compact and Perfect Sets
Proposition 5.2.11: Perfect sets are Uncountable
Proof:
If S is perfect, it consists of accumulation points, and therefore can not be finite. Therefore it is either countable or uncountable. Suppose S was countable and could be written as- S = { x1, x2, x3, ...}
Take one of those elements, say x2 and take a neighborhood U2 of x2 such that closure( U2 ) is contained in U1 and x1 is not contained in closure( U2 ). Again, x2 is an accumulation point of S, so that the neighborhood U2 contains infinitely many elements of S.
Select an element, say x3, and take a neighborhood U3 of x3 such that closure( U3 ) is contained in U2 but x1 and x2 are not contained in closure( U3 ) .
Continue in that fashion: we can find sets Un and points xn such that:
- closure( Un+1 )
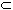 Un
Un
- xj is not contained in Un for all 0 < j < n
- xn is contained in Un
- V =
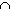 ( closure( Un )
( closure( Un )
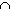 S )
S )
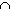 S )
is closed and bounded, hence
compact. Also, by construction,
( closure( Un+1 )
S )
is closed and bounded, hence
compact. Also, by construction,
( closure( Un+1 )
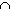 S )
S )
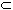 (closure( Un)
(closure( Un)
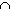 S ).
Therefore, by the above result, V is not empty. But
which element of S should be contained in V ?
It can not be x1, because
x1 is not contained in
closure( U2 ).
It can not be x2 because
x2 is not in
closure( U3 ),
and so forth.
S ).
Therefore, by the above result, V is not empty. But
which element of S should be contained in V ?
It can not be x1, because
x1 is not contained in
closure( U2 ).
It can not be x2 because
x2 is not in
closure( U3 ),
and so forth.
Hence, none of the elements { x1, x2, x3, ... } can be contained in V. But V is non-empty, so that it must contain an element not in this list. That means, however, that S is not countable.
 Interactive Real Analysis
Interactive Real Analysis